Elastic Potential Energy

Objectives:

  • You should be able to calculate the amount of energy associated with a stretching or compressing object
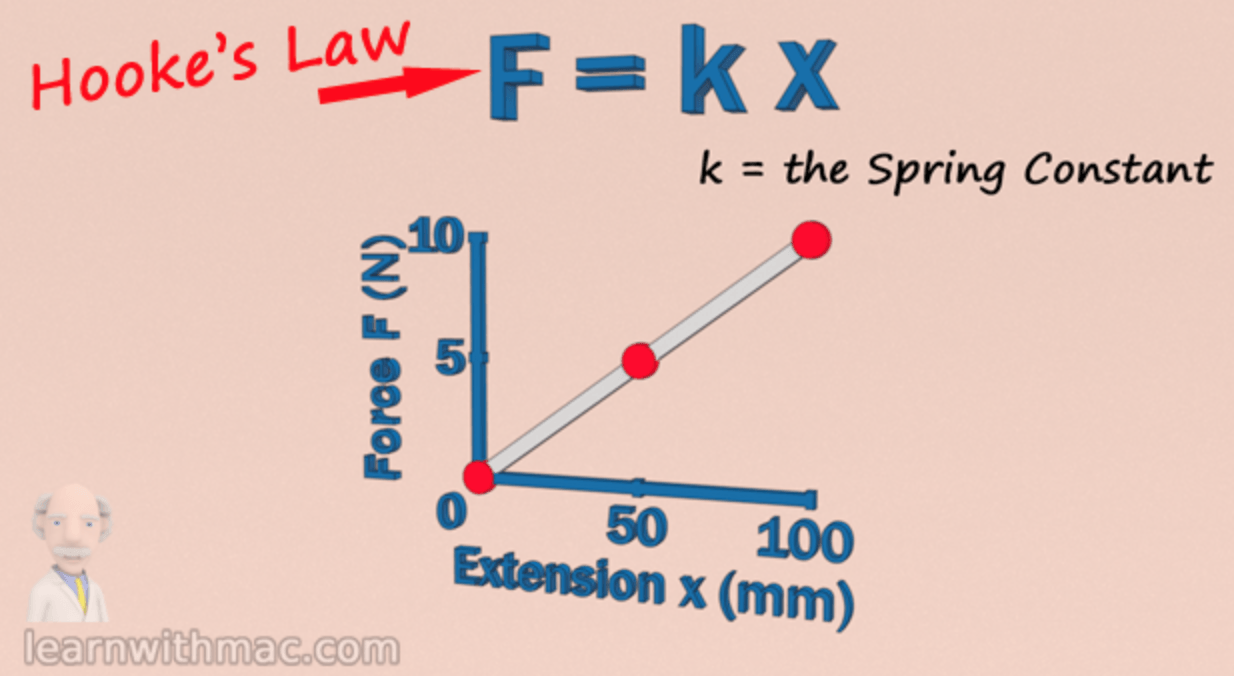
  • The elastic potential energy stored in a stretched of compressed object can be calculated using the equation; E_{e} = 0.5 \times k \times e^{2}

Elastic potential energy is the energy that is stored when an item is either compressed or stretched.

The elastic potential energy of an object is governed by two factors, the stiffness of an object, a property which is referred to as the spring constant, and the amount that the object is stretched by. Both of these factors should make sense since if the spring is not stiff at all, it will require minimal force and minimal energy to stretch it, additionally the further the spring is stretched the more energy is required. The equation for elastic potential energy that links these quantities is:

E_{e} = 0.5 k e^{2}

where;
E_{e} is the elastic potential energy of the object, measure in joules, J
k is the spring constant of the object, measured in newtons per metre, N/m
e is the extension (or compression) from an equilibrium position of the object, measured in metres, m

This equation tells us that for a given spring, the elastic potential energy is proportional to the distance that it is extended by squared:

E_{e} \propto e^{2}

What this means is that if you were to extend a spring by some distance, say e , then to extend it by the same amount again (on top of the original extension) it would require four times as much energy (this assumes that the spring is not changed and so there is the same spring constant, as well as the limit of proportionality is not exceeded):

E_{e} \propto (2 \times e^{2})
E_{e} \propto 4 \times e^{2}
e^{2} \rightarrow multiplies by 4
therefore: E_{e} \rightarrow multiplies by 4

The amount of energy required to extend (or compress) a spring is proportional to the spring constant:

E_{e} \propto k

What this means is that if you were to take a spring that has double the spring constant of another, then the amount of energy required to stretch it by the same amount is double:

E_{e} \propto (2 \times k)
k \rightarrow multiples by 2
therefore: E_{e} \rightarrow multiplies by 2

Energy is a pivotable concept in physics because of the statement that energy is always conserved, in other words it cannot be created nor destroyed. Elastic potential energy is therefore a very significant type of energy (and so is this equation) because anything that is compressed or stretched has an amount of elastic potential energy stored in it that can be calculate using this equation. This has more applications than you can imagine: It enables us to determine how much a spring must be extended to launch a cannonball a certain distance, it tells us how much energy would be required to separate bonds between molecules, it also tells us how much energy is required to fuse particles together (if the bond is treated as a spring holding the particles apart).

Examples of using this equation

  1. A spring, which has a spring constant 8.5 \ N/m , has been stretched 0.30 \ m from its equilibrium position. What is the potential energy that is stored in the spring?
  3. Gabriella jumps into her car and the spring suspension compresses by 2 \ cm . The force she exerts on the spring is 690 \ N . What is the spring constant of the spring?
  4. Continuing on from the previous question, how much elastic potential energy is stored in the spring when compressed by 2 \ cm ?

Solutions

  1. k = 8.5 \ N/m
    e = 0.30 \ m
    E_{e} = 0.5 k e^{2} = 0.5 \times 8.5 \times 0.30^{2}
    $latex E_{k} = 345.6 \ J $
  2. m = 56 \ g = 0.056 \ kg
    v = 1.7 \ m/s
    E_{k} = 0.5 m v^{2} = 0.5 \times 0.056 \times 1.7^{2}
    E_{k} = 0.081 \ J
  3. E_{k} = 0.081 \ J
    m = 56 \ g = 0.056 \ kg (the mass has not changed)
    E_{k} = 0.5 m v^{2} \rightarrow 0.081 = 0.5 \times 0.056 \times v^{2}
    2.89 = v^{2}
    v = 1.7 \ m/s
  4. m = (230 + 80) \ kg = 310 \ kg (total mass is the rider and the bike)
    v = 34 \ m/s
    E_{k} = 0.5 m v^{2} = 0.5 \times 310 \times 34^{2}
    E_{k} = 179,180 \ J = 179 \ kJ

Where doe the equation for elastic potential energy come from?

Click here to find out