4. Einstein's Equation and the Photoelectric Effect : Educating Physics

Objectives:

To understand Einstein’s photoelectric equation and how to arises; $hf = \Phi + K.E_{max}$
To understand the terms work function and threshold frequency
To appreciate the idea that the maximum kinetic energy of the photoelectrons is independent of the intensity of the incident radiation
To understand that rate of emission of photoelectrons above threshold frequency is directly proportional to the intensity of the incident radiation

Einstein’s Photoelectric Equation

As mentioned on the previous page, if an electron absorbs a single photon of a high enough energy then that electron can escape – it would then be called a photoelectron (a photoelectron is exactly the same as an electron, but it has just undergone the photoelectric effect). This photoelectron will then leave the metal plate, the energy it then has would be in the form of kinetic energy. The following equation can then be written showing the conservation of energy:

$E = \phi \ + \ K.E$

where
$E$ is the energy of the photon
$\phi$ is the minimum energy required for an electron to escape the metal and is known as the work function.
$\ K.E$ is the kinetic energy of the photoelectron

Some electrons will have originated from further in the metal and therefore they will have a higher work function to overcome. Therefore the photoelectrons will have a maximum kinetic energy. Additionally, since the energy of a photon can be written as $E = hf$ , we can complete the equation above to give:

$hf = \phi \ + \ K.E_{max}$

The work function, $\phi$ varies depending on the type of metal, zinc has a work function equal to $4.33 \ eV = 6.928 \times 10^{-19} \ J$ . If visible light with a frequency of $5.0 \times 10^{15} \ Hz$ is incident upon a negatively charged zinc plate then we can use the equation above to determine whether electrons will escape the metal and if they do how much kinetic energy they may have, for example:

$hf = \phi \ + \ K.E_{max}$

$6.63 \times 10^{-34} \ \times \ 5.0 \times 10^{15} = 6.928 \times 10^{-19} \ + \ K.E_{max}$

$3.315 \times 10^{-19} = 6.928 \times 10^{-19} \ + \ K.E_{max}$

$K.E_{max} = 3.315 \times 10^{-19} - 6.928 \times 10^{-19}$

$K.E_{max} = -3.613 \times 10^{-19}$

Because this number is a negative value, it tells us that photoelectrons would not be emitted from the plate as the incident photons would not have given them enough energy to overcome the work function (they are $3.613 \times 10^{-19} \ J$ short).

If ultraviolet radiation with a frequency of $1.5 \times 10^{15} \ Hz$ is incident upon a negatively charged zinc plate we can repeat the process above to determine if photoelectrons would now be emitted:

$hf = \phi \ + \ K.E_{max}$

$6.63 \times 10^{-34} \ \times \ 1.5 \times 10^{15} = 6.928 \times 10^{-19} \ + \ K.E_{max}$

$9.945 \times 10^{-19} = 6.928 \times 10^{-19} \ + \ K.E_{max}$

$K.E_{max} = 9.945 \times 10^{-19} - 6.928 \times 10^{-19}$

$K.E_{max} = 3.017 \times 10^{-19}$

Because this number is a positive value, it tells us that photoelectrons will be emitted from the plate.

Now we know that electrons escape and have kinetic energy, the maximum speed at which they can travel can now be determined –The (rest) mass of an electron is $m_{e} = 9.11 \times 10^{-31} \ kg$ :

$K.E_{max} = \frac{1}{2}m_{e}v^{2}$

$\frac{1}{2} \times 9.11 \times 10^{-31} \times v^{2} = 3.017 \times 10^{-19}$

$v^{2} = 6.623 \times 10^{11}$

$v = 8.138 \times 10^{5} ms^{-1}$

$v = 8.1 \times 10^{5} ms^{-1} (2s.f)$

This tells us that this UV light striking a zinc plate will emit a photoelectron with a velocity of $8.1 \times 10^{5} \ ms^{-1}$ .

In order for photoelectrons to be emitted, the incident photon needs to have at the very least the energy required to overcome the work function. If the photon had a very specific frequency known as the threshold frequency $f_{th}$ , such that it has energy that matches the work function energy we can write:

$hf_{th} = \phi$

Taking the zinc plate with a work function of $4.33 \ eV = 6.928 \times 10^{-19} \ J$ , we can determine the absolute minimum intensity required for photoelectrons to be emitted:

$6.63 \times 10^{-34} \ \times f = 6.928 \times 10^{-19}$

$f = \frac{6.928 \times 10^{-19}}{6.63 \times 10^{-34}} = 1.045 \times 10^{15} \ Hz$

So for a photoelectron to be emitted from a plate: $E \ > \ \phi$ .

Using the wave speed equation, the minimum wavelength of the incident photon required for a photoelectron to escape can also be determined:

Using $E = hf$ and $c = f\lambda$

$E = \frac{hc}{\lambda}$

The minimum energy required needs to be greater than the work function $E \ > \ \phi$ , so:

$\frac{hc}{\lambda_{th}} \ > \ \phi$

where $\lambda_{th}$ is the threshold wavelength

If a pair of parallel plates are connected across an e.m.f. source, as shown in the image to the left. One of these plates would become and anode and the other a cathode.

With light, with a frequency equal to the threshold frequency, being incident on the cathode, electrons would absorb the photons and gain enough energy to overcome the work function;

$f = f_{th}$ and therefore $E_{photon} = \phi$

This would result in a photoelectron being on the verge of escaping the cathode.

Since electrons are negatively charged, they would then be repelled by the cathode and attracted to the anode (they will be in an electric field). This would result in the electron being accelerated across the potential difference between the plates.

If the call provides an e.m.f. of 1 V, then using Kirchhoff’s second law the potential difference across the cathode and anode will also be 1 V.

This electron will then accelerate across this potential difference of 1 V. If this is done inside an evacuated tube (such that none of the electrons gained kinetic energy is lost, the amount of energy that the electrons gain just before they collide with the anode can be determined:

$V = \frac{W}{Q}$

where
V is the potential difference across the plates
W is the work done on accelerating the charged particle
Q is the charge of the particle, in this case an electron, so $Q = e$

Rearranging this gives:

$W = QV$
$W = e \times 1 V$
$W = 1 \ eV$

This is the definition of the electronvolt.

If the voltage were to change to say 230 V, then the energy that any electron would gain would be $W = 230 \ eV$

For more information try this video clip:

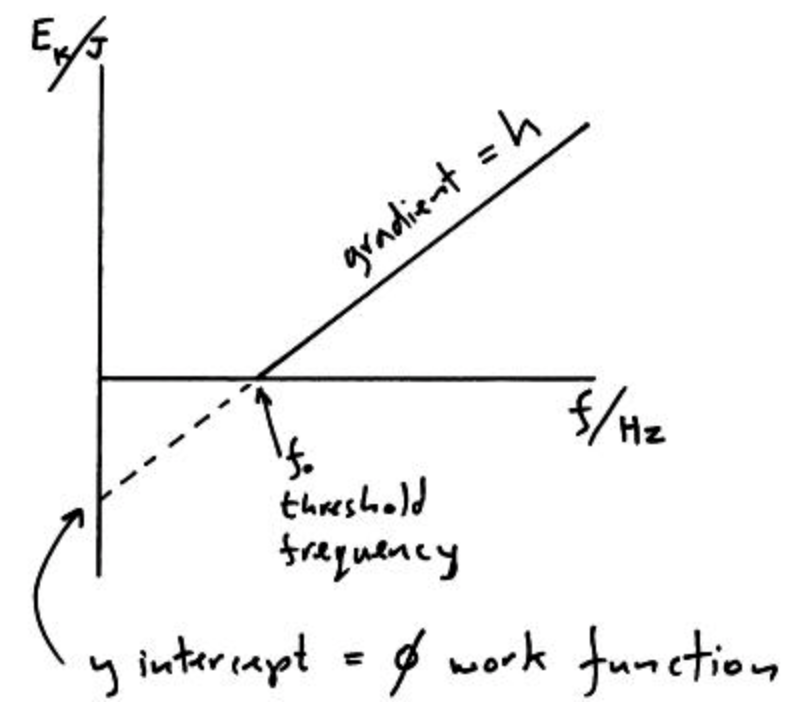
Using the circuit setup show above we can tweak Einstein’s photoelectric equation:

$hf = \phi \ + \ K.E_{max}$

If the incident photons only provide just enough energy to overcome the work function, then the energy that the photoelectron will gain by the time they reach the anode will be equivalent to the work done due to the potential difference ( $W = eV$ ). Therefore we can write: